GENERAL CHEMISTRY TOPICS

Oxidation-reduction reactions

Definitions of oxidation, reduction and related terms. Characteristic oxidation-reduction ("redox") reactions. Oxidation states (a.k.a. oxidation numbers). Rules for assigning oxidation states. Using oxidation states to identify redox reactions.

---

Oxidation-reduction (or "redox") reactions are a very large class of chemical reactions in which both oxidation and reduction necessarily occur.

An oxidation is defined as loss of electrons in the course of a chemical reaction. If a species gains electrons, it is undergoing a reduction. Since electrons are "conserved" in a chemical reaction (they are not created or destroyed), one chemical species' loss is another's gain. Thus, a reduction cannot occur with a corresponding oxidation, and vice-versa - a kind of yin-yang principle. The term "redox" also nicely encapsulates how inextricably tied together oxidation and reduction are in reality. Other terminology used in discussing redox chemistry: A chemical species that gets reduced is acting as an oxidizing agent, or oxidant, while the species undergoing oxidation is acting as the reducing agent, or reductant.

Oxidation state (or oxidation number) is a bookkeeping device employed by chemists to help them classify and understand chemical reactions. The simplest way to interpret oxidation number is to think of it as the number of electrons lost or gained by an atom (compared to its neutral, uncombined form) when it reacts to form ions or molecules. Consider first the case of ions. For monatomic ions, such as Na⁺ or Cl⁻, the oxidation number is the same as the charge, +1 and −1, for the sodium cation and chloride anion, respectively. More generally, in calculating an oxidation number, we treat any differences in electronegativity between covalently bonded atoms as if the bond is actually ionic. That means both electrons are counted as belonging to the more electronegative atom. Note how this differs from formal charge: In computing formal charge, we treat electrons in covalent bonds as equally shared, despite differences in electronegativity. But like formal charge, the sum of the oxidation numbers for each atom in a formula or Lewis structure for a molecular or ionic species must sum to the net charge of that formula or Lewis structure (zero for a molecule). We will soon see that assignment of oxidation numbers and following how they change in a chemical reaction allows us to recognize redox reactions and determine the stoichiometry of the electron transfer occurring.

It is easy to recognize any reaction featuring an uncombined, neutral element as a redox reaction. Some examples are

CH₄(g)  +  2 O₂(g)  →   CO₂(g)  +  2 H₂O(l)

Fe(s)  +  O₂(g)  →  Fe₂O₃(s)

2 Na(s) + Cl₂(g)  →  2 NaCl (s)

which represent combustion of methane, rusting of iron, and the formation of salt. In the first, the elemental form of oxygen reacts, with the oxygen ends up in carbon dioxide and water. In the second, two uncombined elements, a metal and nonmetal react to form an ionic compound. In the third example, an ionic compound is again formed from combination of elemental forms (metal and nonmetal), but oxygen is not involved, illustrating that chemists have generalized the original concept of oxidation. What if there are no uncombined, nonionic forms of elements in a chemical reaction? Is it still possible to have a redox reaction? The answer is yes, and the following reactions provide examples:

8 H⁺(aq)  +  2 NO₃⁻(aq)  +  3 Sn²⁺(aq)    →    4 H₂O(l)  +  2 NO(g)  +  3 Sn⁴⁺(aq)

16 H⁺(aq)  +  3 C₂H₅OH(aq)  +  2 Cr₂O₇²⁻(aq)   →   11 H₂O(l)  +  3 CH₃CO₂H(aq) +  4 Cr³⁺(aq)

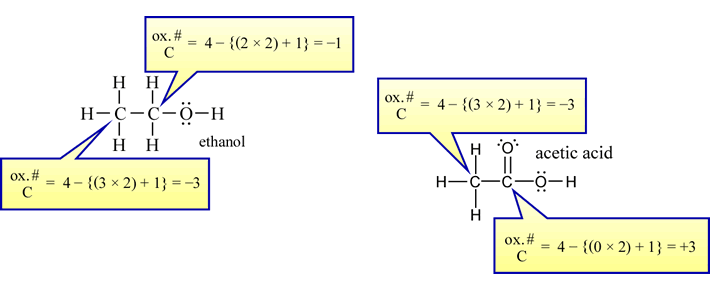
The first reaction clearly shows the tin(II) ion being oxidized to the tin(IV) ion. The accompanying reduction is less easily picked out. The second reaction, which is the basis for the Breathalyzer™ test, might be suspected of being a redox reaction by noting that chromium (Cr) appears in two different ionic forms, and that ethanol (C₂H₅OH) is being converted to a compound (CH₃CO₂H, acetic acid) containing more oxygen and less hydrogen in combination with a fixed number (two) of carbon atoms.

In order to identify in all cases whether a given reaction is an oxidation-reduction reaction, and to also determine the electron stoichiometry of a redox reaction, we use a set of bookkeeping rules to assign oxidation states (or oxidation number) to each individual atom in the molecules and ions involved in a given chemical reaction. We look for changes in oxidation states, and when oxidation states change in a reaction we can say the reaction is a redox reaction. If the oxidation state of an element decreases in a reaction, it is undergoing reduction. In cases where oxidation state of an element increases, that element is being oxidized.

Rules for assigning oxidation states

The rules for assigning oxidation states are fairly simple. The following formulation is based on that given by Tro (Ref. 1, below).

1. The oxidation state of an atom in an elemental form is zero.
2. The oxidation state of an atom in monatomic ionic form is equal to the charge on the ion.
3. The sum of all oxidation states in a molecule, polyatomic ion, or formula unit is always equal to the net charge.
4. A hierarchical series of oxidation states for elements, based fundamentally on their valences and the electronegativity scale, is used to assign oxidation states for individual elements as follows:
   1. Group I elements (Li, Na, K,...) always have a +1 oxidation state in their compounds.
   2. Group II elements (Be, Mg, Ca,...) always have a +2 oxidation state in their compounds.
   3. Fluorine always takes on the oxidation state of −1 in its compounds.
   4. If not dictated otherwise by the above, hydrogen will have an oxidation state of +1 in its compounds.
   5. Unless dictated otherwise by the above rules, oxygen will have an oxidation state of −2 in its compounds.
   6. If not determined already by the rules, other elements will have the following oxidation states (in order of precedence): halogens besides fluorine (Cl, Br, I), −1; sulfur (S) −2, nitrogen (N) −3.
   7. Assignment of oxidation state for any remaining elements not yet assigned should in most cases be possible by application of rule 3.

Examples of assigning oxidation states

Let's work through a number of examples that demonstrate the use of the above set of rules.

• Cl₂. Since the oxidation state of an atom in an elemental form is zero (rule 1), the oxidation state (or oxidation number - we can abbreviate this as "ox.#") for chlorine in diatomic molecular chlorine is zero. Note that oxidation state is formally assigned to each atom in a molecule or polyatomic ion. So each of the two chlorine atoms in Cl₂ is assigned the value zero. The sum of the two oxidation numbers is also zero, the net charge of the neutral species (rule 3 is and must always be satisfied).
• Fe³⁺. Since the oxidation state of an atom in monatomic ionic form is equal to the charge on the ion (rule 2), the oxidation number of an iron(III) cation is straightforwardly assigned an oxidation state of +3.
• CuCl₂. We recognize this as the ionic compound copper(II) chloride. If we simply treat this case as a neutral formula unit made of of three monatomic ions, we can apply rule 2 again and assign oxidation state of +2 to the Cu²⁺ on, and −1 to each of the chloride ions. Alternatively, application of rule 3 (which must always be satisfied) following rule 4f leads to each Cl being assigned −1 and Cu becomes +2. Assignment of oxidation states to atoms in ionic compounds composed solely of monatomic ions mimics the procedure for writing neutral formula units given predictable ionic charges.
• H₂O, H₂, LiH. Here we highlight the differing oxidation states an element can take on in different contexts. In the hierarchy of rule 4, the first rule that applies specifically to hydrogen is rule 4d. This is the appropriate rule for H in H₂O, so each hydrogen will have its most common oxidation state of +1, which in turn dictates by rule 3 that the oxygen will have an oxidation state of −2. This also agrees with the most common oxidation state of the latter, as expressed in rule 4e. For H₂, rule 1 applies, and each hydrogen has an oxidation state of zero in this case. Finally, the ionic compound lithium hydride shows the hydrogen in a less typical −1 oxidation state, which follows by application of rule 4a, which assigns oxidation state of +1 to the group 1A metal lithium (also its predictable ionic charge). Note that rule 4a take precedence over rule 4d. This, plus rule 3, forces the −1 oxidation state for hydrogen.
• CH₄. This case is similar to that of H₂O above, in that rule 4d applies to confer upon hydrogen its most common oxidation state of +1. However, there is not a rule for carbon. This means that the oxidation state of carbon must be determined by rule 3. We will see that carbon takes on a full range of oxidation states in its compounds. Here rule 3 determines that the oxidation number of carbon is −4 in methane, which is its most reduced form (remember, a reduction is literally a "reduction" of oxidation state) of all one-carbon molecules containing hydrogen and/or oxygen.
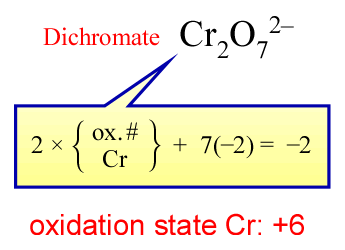• Cr₂O₇²⁻. This is a more difficult but also instructive case. Here, rule 4e applies and gives oxygen its most common oxidation state of −2. There is not a rule for chromium, so its oxidation state will be determined by rule 3. An algebraic expression can be written that represents the fulfillment of rule 3. This is shown in the figure at right, leading to the assignment of oxidation state +6 to each chromium in dichromate.

Exercise: Assign oxidation states to carbon in carbon dioxide, formic acid (CH₂O₂), formaldehyde (CH₂O), and methanol (CH₃OH).

Identifying oxidation-reduction reactions using oxidation states

Applying these rules to the two previous reactions shows that the oxidation states of H and O do not change from their usual values (+1, −2, respectively) in either case. In the first reaction, tin is oxidized (its oxidation state is +2 on the reactant side, and +4 on the product side, by rule 2), while the oxidation state of N in the nitrate anion is +5, and in nitrogen monoxide it is +2 (by rules 3 and 4), so nitrogen undergoes reduction. In the second reaction, chromium is reduced - its oxidation state is +6 in the dichromate (Cr₂O₇²⁻) anion (by rules 3 and 4) on the reactant side, and is +3 (by rule 2) on the product side. The two carbon atoms in ethanol can be assigned an oxidation state of −2 (it is actually an average in this case - two atoms of the same element can have different oxidation states depending on how they are bonded in the molecule or ion under consideration, but that need not concern us further here), while in the product acetic acid, we arrive at an oxidation state of zero for carbon. We see that the organic compound ethanol is being oxidized to acetic acid by the oxidizing agent dichromate.

Lewis structures and oxidation state

For Lewis structures, the assignment of oxidation states to atoms can be made on the basis of the following condensed rule: The oxidation state of an atom in a Lewis structure is the number of valence electrons in the neutral atom minus the total count of lone pair electrons, electrons in bonds to less electronegative atoms, and one half the electrons in bonds to atoms with the same electronegativity (usually atoms of the same element). To illustrate this rule, oxidation states can be assigned to the individual atoms in the one- and two-carbon molecules also containing hydrogen and/or oxygen.

 

For two-carbon molecules, it emerges that assignment of oxidation state according to the set of rules above to polyatomic species with two or more atoms of the same element strictly by formula yields an average oxidation state for the atoms of that element. The oxidation states assigned to atoms in a Lewis structure shows how they differ from an average based on a formula.