BIOCHEMISTRY TOPICS

Michaelis-Menten equation

Assmptions underlying the mechanistic model that leads to the Michaelis-Menten equation.

---

Consider the simple model for the enzymatic catalysis of a unimolecular reaction converting a substrate S to a product P, shown below. We will derive an expression for the initial rate of the enzyme catalyzed reaction represented by this model, which we will call the Michaelis-Menten equation.

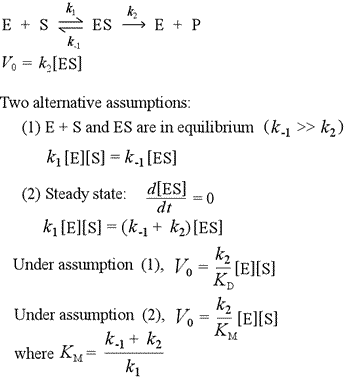
Three rate constants are defined in this model: the forward rate constant k₁ for the formation of the enzyme-substrate (ES) complex from free enzyme and unbound substrate; the reverse rate constant k₋₁ for the dissociation of ES back to E + S; and the rate constant for the catalytic step, k₂, i.e. the unimolecular rate constant for the breakdown of ES to E + P. Note that in the absence of a catalytic step, ES and E + S would reach an equilibrium, with an equilibrium constant K_eq = k₁/k₋₁. We define a dissociation constant, K_d, which is the inverse of K_eq. Binding affinities are often measured or reported in terms of K_d. A lower K_d means a tighter complex; a higher K_d means a weaker complex. The initial rate V₀ of the forward reaction is fundamentally dependent on the concentration of ES, and is given by the equation V0 = k₂[ES]. We can make two alternative assumptions to obtain an expression to substitute for [ES].

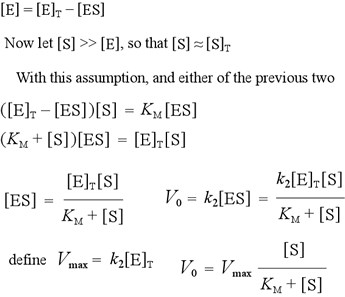
To continue with the derivation of the rate equation for our simple kinetic model, we note the relation between the concentrations of free enzyme, the enzyme-substrate complex, and the total enzyme. In the case where substrate is present in significant excess over enzyme - a reasonable condition since enzymes accelerate reactions in amounts substoichiometric to substrate - the analysis is simplified. We can then solve explicitly for [ES] in terms of experimental "knowns" - the total enzyme and total substrate concentrations - and the parameter K_M. Then we use the expression V₀ = k_cat[ES] to express the measurable initial reaction rate in these terms, plus the additional parameter V_max.

An important point: Although this equation was derived using a particular simple mechanism, it is valid for many more complex mechanisms. It reproduces the saturation kinetics characteristic of enzyme-catalyzed reactions.

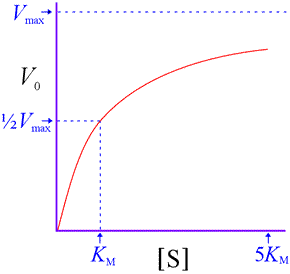
That is, V₀ approaches a limit of V_max as substrate concentration increases. We also see that K_M represents a substrate concentration at which V₀ reaches one-half V_max. (Substitution of ½V_max for V₀ in the above equation and solving for [S] shows that [S] = K_M. ) Note that [S] must be quite large before V₀ becomes close to V_max . For instance, at [S] = 5K_M, the initial rate is only 83% of V_max.