BIOCHEMISTRY TOPICS
Equilibrium and free energy
Important results of chemical thermodynamics. The variation of free energy with composition for a chemical reaction.
Applications of the free energy function
- Standard free energy change (ΔG°) can be used to calculate an equilibrium constant
- Actual concentrations of reactants and products for non-equilibrium conditions can be used to
calculate ΔG, which is useful for:
- predicting direction of reaction (approach to equilibrium)
- quantifying the energy available for non-expansion work (at constant T, P)
Let us introduce the key results of chemical thermodynamics by considering a hypothetical chemical reaction that takes place under conditions of constant pressure and temperature.
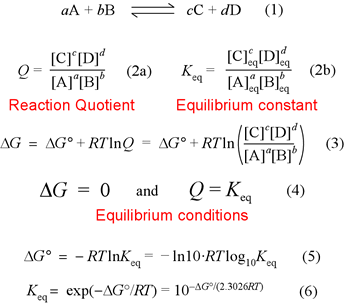
Representing a generalized chemical reaction of two reactants A and B, with stoichiometric coefficients a and b, and two products C and D, with stoichiometric coefficients c and d, the expressions for the equilibrium constant Keq and reaction quotient Q (expressing the law of mass action) are obtained by taking terms for product concentrations (M) and placing them in the numerator, and likewise terms for the reactant concentrations go to the denominator. Each term is raised to the power of its stoichiometric coefficient.
The equation labeled (3) is the fundamental relation. It says that the free energy change for a reaction can be calculated under any composition of a mixture of reactants and products. Now, since the free energy G is a chemical potential energy function for the system consisting of this mixture of reactants and products, if conditions permit their facile interconversion the composition will change until the minimum value of free energy is attained, which is exactly the equilibrium composition. In other words, at the minimum of free energy attainable by this system, Q = Keq, and we assign ΔG = 0 under these conditions. See the next figure below, which illustrates this for a specific chemical reaction and value of Keq. By analogy to a mechanical system, there is no driving force for composition to change at equilibrium. For a very small change in composition Δc proceeding from the equilibrium composition, ΔG ≈ (dG/dc)Δc = 0 since the slope of the tangent to the free energy vs. concentration curve is zero at that point.
Finally, equation (3) and the equilibrium condition just discussed leads to a relation between what is called the standard free energy change, ΔG°, and the equilibrium constant Keq.
ΔG° = −RTlnKeq
This, along with alternate forms of this relation, is shown in equations (5) and (6). Note that ΔG° must correspond to conditions for which Q = 1.
We have assumed for simplicity that all the species in the chemical equation are solutes in dilute aqueous solution. There are cases in which terms for reactants or products are not included - usually arising for heterogenous systems in which reactant or product is a pure liquid or solid. It is also worth noting that the concentration terms in the square brackets are treated - from a thermodynamic point of view - as unitless numbers representing the molar concentrations of the corresponding species. In other words, the concentration of each species, expressed in units of molarity (M) is divided by the reference concentration 1 M (exactly). This may seem like a minor issue, but its importance looms if we decide (as we do in defining biochemical standard state) to set a different reference concentration for a species (setting pH 7 or [H+] = 1 × 10−7 M as the reference concentration for H+).
Finally, while we routinely substitute concentrations in evaluating Keq or Q, this is only valid in the limit as concentrations approach zero. In order to correct for this nonideal behavior, the quantity activity is introduced. Activities include a correction factor applied to concentration that becomes more significant as concentrations increase. This correction factor also becomes more prominent as species become charged or increase in charge. Often we are content to ignore the inaccuracies that are inevitable in just using concentrations, which can be adequate as long as the solutions are sufficiently dilute.
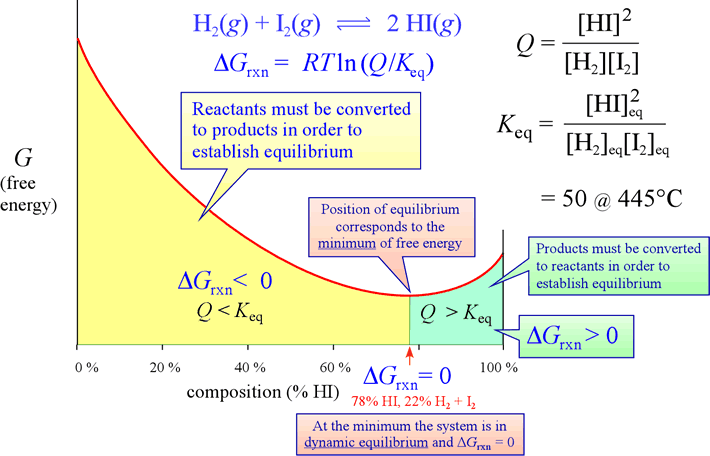
The variation of free energy with composition for a chemical reaction
Given the equations of chemical thermodynamics just discussed, the relative free energies as reactants and products vary in composition can be calculated. In the specific example of the gas phase reaction between diatomic hydrogen and diatomic iodine to form hydrogen iodide, the equilibrium constant is 50 at a temperature of 445 °C. At this temperature the reactants and products can readily interconvert to establish dynamic equilibrium. For an equal number of moles of hydrogen and iodine, in principle the composition can vary between 0% HI (100% H2 + I2) and 100% HI (0% H2 + I2). If such a system had a composition of 1 mol each of H2 and I2, a net conversion of reactants to products would be thermodynamically favorable (free energy decreases as HI forms). Conversely, for a composition of 2 mol HI, the net conversion of products to reactants would be thermodynamically favorable (free energy decreases as HI reverts to H2 + I2). However, in either case once the minimum of free energy is attained, no further change in composition would be observed. These two cases can be also viewed in terms of the comparison of the values of Q and Keq. In the figure below, we can think of Q as a vertical line that we can slide back and forth, varying the composition and reading the relative free energy of the system at that composition. At any given composition, the actual free energy change "driving" the reaction in one direction or the other under those conditions, is given by* ΔGrxn. If conditions are such that ΔGrxn < 0 (Q < Keq), then net conversion of reactants to product is favorable (lowers free energy}. If ΔGrxn > 0 (Q > Keq), then net conversion of product to reactants is favorable (lowers free energy; note the negative sign for free energy change applies to the reverse reaction) under these conditions. At Q = Keq, the vertical line intersects the minimum of the free energy curve, and ΔGrxn = 0. Net conversion in either direction is unfavorable (increases free energy).
(*What we label here as ΔGrxn is ΔG in equation (3) above, in which −RTlnKeq was substituted for ΔG° so that
ΔG = −RTlnKeq + RTlnQ = RTln{Q/Keq}
Calculating ΔG°rxn for chemical reactions
The free energy change for a chemical reaction, ΔG°rxn, can be calculated using the relation ΔG°rxn = ΔH°rxn − TΔS°rxn or by using ΔG°f values.
We demonstrate here the calculation of the free energy of a chemical reaction by using ΔG°rxn = ΔH°rxn − TΔS°rxn . We can calculate ΔH°rxn by a stoichiometrically weighted sum of ΔH°f values obtained from tables of thermodynamic data. Similarly, ΔS°rxn is calculated using S°T, m values from thermodynamic tables.
N2(g) + 3 H2(g) = 2 NH3(g)
where the reaction is carried out at 298.15 K (25.00 °C). (To see how the ΔH°rxn and ΔS°rxn values wer calculated for this example, see the enthalpy and entropy topics pages, respectively.
ΔH°rxn = − 92.22 kJ and ΔS°rxn = − 198.53 J/K
per mol of N2 that reacts. Let's just put these result together to find that
ΔG°rxn = ΔH°rxn − TΔS°rxn = − 92.22 kJ − (298.15 K) ( − 198.53 J/K)
ΔG°rxn = − 92.22 kJ − ( − 59.192 kJ) = − 33.03 kJ
Related topics pages: